ADRE Grade IV Exam Mathematics MCQ with Answers

The ADRE Grade IV Exam Mathematics MCQ set is designed to help candidates prepare for the upcoming Assam Direct Recruitment (ADRE) Grade IV Exam, scheduled for 29th October. This set includes 20 multiple-choice questions (MCQs), specifically focused on Maths skills.

Each question comes with a detailed explanation to help candidates understand the Mathematics behind the correct answer. This resource offers a realistic simulation of the exam by aligning with the official syllabus and difficulty level.

By practicing these questions, candidates can improve their problem-solving efficiency, critical thinking, and overall confidence, increasing their chances of success in the ADRE Grade IV exam.

ADRE Grade IV Exam Mathematics MCQ with Answers

1. In a row of boys, Srinath is 7th from left and Prabin is 12th from right. If they
interchange their positions, Srinath becomes 22nd from the left. How many
boys are there in the row?

Solution:

Srinath is 7th from the left and Prabin is 12th from the right. After they switch places, Srinath becomes 22nd from the left.

The total number of boys is: 22+12−1=3322 + 12 – 1 = 3322+12−1=33

Thus, there are 33 boys in the row, which is option (C).

2. If 72 96 = 6927, 58 87 = 7885, then 79 86 = ?

Solution:

The pattern is reversing the two numbers:

• 72 x 96⇒6927
• 58 x 87⇒7885

Reverse them to get 6897, which is option (C).

3. If ‘÷’ means ‘addition’, ‘+’ means ‘subtraction’, ‘–’ means ‘multiplication’ and ‘x’ means ‘division’, then the value of 18 ÷ 12 x 4 – 5 is

Solution:

Given the new meanings of the operations:

• ‘÷’ means addition (+),
• ‘x’ means division (÷),
• ‘–’ means multiplication (×),
• ‘+’ means subtraction (−),

Now, rewrite the expression 18 ÷ 12 x 4 – 5 using these new meanings: 18+12÷4×5

Step-by-step solution:

1. 12 ÷ 4 = 3
2. 3 × 5 = 15
3. 18 + 15 = 33

So, the value of the expression is 33, which is option (D).

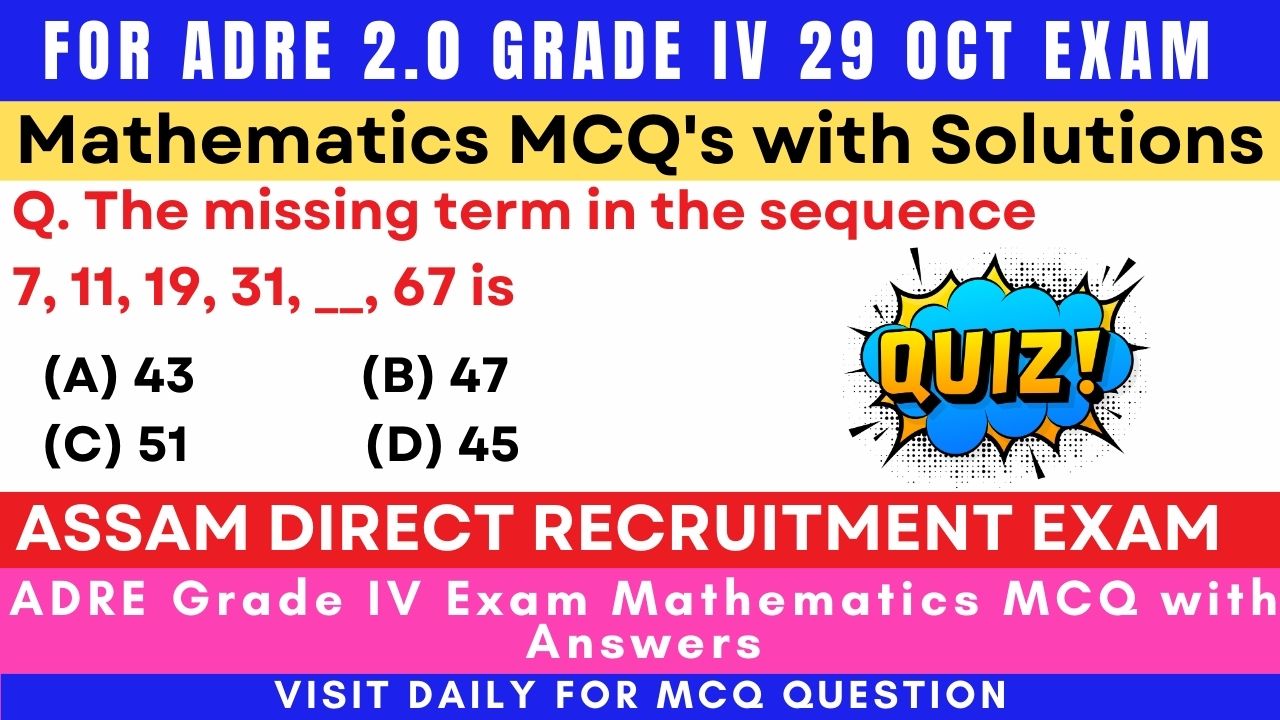
4. The missing term in the sequence 7, 11, 19, 31, __, 67 is

Solution:

The differences between consecutive terms are:

• 11−7=4
• 19−11=8
• 31−19=12

It shows that the differences increase by 4 each time.

So, the next difference should be 12+4=16

Thus, the missing term is:

31+16=47

Therefore, the missing term is 47, which is option (B).

5. The Chairman enters the Assembly Hall 10 min before 12:30 hours to conduct the interview. The Chairman was 20 min earlier than the MD. The MD was 30 min late as per time fixed for the interview. The time fixed for the interview was :

Solution:

• The Chairman enters 10 minutes before 12:30, so he arrives at 12:20.
• The Chairman is 20 minutes earlier than the MD, meaning the MD arrives at 12:40.
• The MD is 30 minutes late, so the interview was originally scheduled for 12:10.

Thus, the time fixed for the interview is 12:10 hours (option D).

6. The 8th term of the sequence 2, 6, 18, 54, …… is

Solution:

The sequence is 2,6,18,54,… with a common ratio of 3.

Using the formula for the n-th term:

T_n=2×3ⁿ⁻¹

For the 8th term:

T₈=2×3⁷=4374

Thus, the 8th term is 4374, (option B).

7. Two numbers are respectively 20% and 50% more than a third number. The ratio of the two numbers is

Solution:

Let the third number be x.

• First number: 1.2x (20% more)
• Second number: 1.5x (50% more)

The ratio of the two numbers is:

1.2x/1.5x=4/5

So, the ratio is 4 : 5 (option C).

8. The simple interest earned by ` 4,000 in 18 months at 12% per annum is

Solution:

Using the simple interest formula:

SI=P×R×T/100

For P=4000, R=12%, and T=1.5 years:

SI=4000×12×1.5/100=72000/100=720

The simple interest earned is ₹720 (option B).

9. When a number is divided by 893 the remainder is 193. If the same number is divided by 47, the remainder will be :

Solution:

Given N=893k+193

1. Find 193mod  47:

193÷47≈4⇒193−(4×47)=5

2. Find 893mod  47:

893÷47=19⇒893−(19×47)=0

Thus: N mod  47=(0+5) mod  47=5

The remainder when divided by 47 is 5 (option C).

10. A hostel has 120 students and food supplies are for 45 days. If 30 more students joined the hostel, then how many days the hostel will run with the existing food ?

Solution:

Total student-days of food:

120×45=5400 student-days

New number of students:

120+30=150

Days food will last:

5400/150=36

So, the food will last for 36 days (option C).

11. A 20 m long ladder is leaning on a vertical wall. It makes an angle of 30° with the ground. The height of the point the ladder touches wall is

Solution:

sin(30° )=height​/20

Since sin⁡(30∘)=0.5

height=0.5×20=10 m

Answer: (A) 10 m.

12. A wheel can cover a distance of 22 km in 1000 rounds. The radius of the wheel is

Solution:

A wheel covers 22 km in 1000 rounds. The distance per round is:

22000 m.1000=22 m

Using the circumference formula 2πr=22, solve for r

r=22/​2π≈3.5m

Answer: (D) 3.5 m.

13. In a school with 10 teachers, one retires and immediately a new teacher of age 25 years joins. As a result, the average age of the teacher reduces by 3. The age of the retired teacher is

Solution:

Let the original average age be x. The new average is x−3.

Using total age:

10x−age of retired teacher+25=10x−30

Solve for the age of the retired teacher:

Age=25+30=55

Answer: (A) 55 years.

14. The ratio of the number of boys and girls in a school of 720 students is 7 : 5. How many more girls should be admitted to make the ratio 1 : 1 ?

Solution:

Boys = 420, Girls = 300 (from the 7:5 ratio).

To make the ratio 1:1, we need 420 girls.

So, 420−300=120 more girls are needed.

Answer: (B) 120.

15. Shyam stored ` 35 in the form of 1 rupee coin and 50 paise coins in the ratio 2 : 3. The number of 50 paise coins are :

16. If the sum of five consecutive numbers is 190, then the lowest number amongst them is

Let the five consecutive numbers be x, x+1, x+2, x+3, x+4x,

The sum of these numbers is:

x+(x+1)+(x+2)+(x+3)+(x+4)=190

Simplifying the equation:

5x+10=190

Subtract 10 from both sides:

5x=180

Now, divide by 5:

x=36

Thus, the lowest number is 36.

Answer: (D) 36.

17. A 100 metre long train moving in a uniform speed of 20 m/sec crosses a bridge of length 1 km. The time taken by the train to cross the bridge is

Solution:

To find the time taken by the train to cross the bridge, we first need to determine the total distance the train travels while crossing the bridge. This distance is the sum of the length of the train and the length of the bridge.

1. Length of the train: 100 meters
2. Length of the bridge: 1 km = 1000 meters
3. Total distance: 100 m+1000 m=1100 

Now, we can use the formula for time:

Time=Distance/Speed

Substituting the values:

Time=1100 m/20 m/sec=55 seconds

The time taken by the train to cross the bridge is 55 seconds.

Answer: (C) 55 seconds.